Jim Whitney Economics 250

Homework exercises #20: Key
Due: __________________________

Circle section: 12:50 / 1:55

 Name: ______________________________
     
 
The Pimax Company is operated as a profit-maximizing monopoly. Its total cost function is:
    TC = 294 + Q2 where TC = total cost in dollars, Q = output in units,
and its (inverse) demand function is:
    P = 84 - 2.Q where P = price of output in dollars.

1.

a. (1) Profit-maximizing output:   14
(2) Price: 56
(3) Profits:  294
(4) MC:  28 
(5) ATC:  35 
    Profit max => set MR = MC
    TR = PQ = (84-2Q)Q = 84Q-2Q2 => MR = 84 - 4Q
    TC = 294 + Q2 => MC = 2Q
    (1) MR=MC => 84-4Q = 2Q => Q = 14
    (2) P = 84 - 2Q = 84 - 2(14) => P = 56
    (3) Profits = TR - TC = 56.14 - (294 + 142) = 784 - 490 => Profits = 294
    (4) MC = 2Q = 2(14) => MC  = 28
    (5) ATC = TC/Q = 490/14 => ATC=35 (ATC>MC=>ATC falling at Q=14)
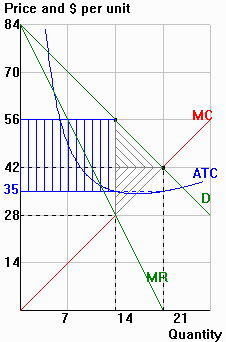b. Sketch Pimax's demand, marginal revenue, marginal cost and average cost curves. Indicate in your diagram: (1) the efficient output level; (2) Pimax's profit-maximizing output level and profits; and (3) the welfare loss at Pimax's output level
    (1) Efficient Q=21; (2) Qm = 14; shaded box = profits; (3) shaded triangle = welfare loss
     Answer key checklist: (1) Did you make MC a straight line starting from the origin? (2) Did you make ATC rise when MC>ATC? (3) Did you use ATC at Qm for the bottom of your profits box?
 
2. Suppose you are directed by the government to regulate Pimax's prices. If your goal is economic efficiency:
a. (1) What price ceiling will you set?   P=42
(2) How much will Pimax produce? Q=21
(3) How large will its profits be? 147
    (1) Efficiency => P = MC => 84 - 2Q = 2Q => Q = 21; P = 84 - 2(21) => P = 42
    (2) Pimax will produce 21--up to Q=21, MR=P=42; and at Q=21, MC=2·21=42. So MR=MC at Q = 21.
    (3) Profits = 4221 - (294 + 212) => Profits = 147
b. Can you recommend a policy to eliminate the profits from part a while not interfering with Pimax's output decision under your price regulation? Explain.
    To get rid of Pimax's residual profits, charge a $147 lump sum tax, say a franchise fee. This will act as a fixed cost, raising Pimax's average costs without affecting its marginal costs.
 

 

3.

(from Frank, Ch.12) What effect will the imposition of a 50 percent tax on economic profit have on a monopolist's profit-maximizing price and output levels?

    A 50% profits tax has no effect. The same output and price athat maximize profits also maximize profits/2.
    1/2(TR - TC) = 1/2*TR - 1/2*TC. p-max => 1/2*MR = 1/2*MC => MR=MC, same condition as without the profits tax.
 
4. Consider the following information about the demand for a particular textbook (P=dollars and Q=1000s of books):
            P = 20 - 0.2.Q
            MC = 6 + 1.68.Q
a. The author collects a royalty equal to 20% of gross receipts (total revenue) on sales of the book. What quantity and price does the author prefer? (Hint: the author's goal is to maximize 20% of TR.)
(1) Q= 50 (thousand)
(2) P= $10

    Maximizing 20% of TR => maximize TR:
    TR = P·Q = (20 - .2·Q)·Q = 20·Q -.2·Q2
    (1) MR = 20 - .4·Q = 0 => Q = 50
    (2) P = 20 - .2(50) = 10

 
b. What quantity and price does the publisher prefer? (Hint: the publisher's profits equal 80% of TR minus TC.)
(1) Q= 5 (thousand)
(2) P= $19

    The publisher wants to maximize its profits:
    TRpub = .8·TR, since 20% of TR goes to author.
    TRpub = .8·(20·Q-.2·Q2) = 16·Q - .16·Q2
    MR = 16 - .32·Q; MC = 6+1.68·Q => Q = 5
    P = 20 - .2·(5) = 19